The Calculus of Consent: Logical Foundations of Constitutional Democracy

We shall now examine the contributions that modern game theory can make toward an analysis of simple majority voting. In one sense we shall be discussing the same problems considered in Chapter 10, but we shall use here a slightly different set of analytical tools. As will become evident to those who are even moderately sophisticated in the field, our constructions will be reasonably elementary. Our purpose is, however, not that of making any contribution to game theory itself, but rather that of applying the relevant theory to our particular problems.*21

The application of game theory to majority voting is relatively straightforward and simple, but the limited extent to which game theory can be helpful for our purposes should be acknowledged at the outset. Most of the refinements in this theory have been developed in the analysis of two-person, zero-sum games. Quite clearly, the analysis of such games will not take us very far in predicting the outcomes of simple majority voting rules in the political process. For assistance here, we must look to the developments in the theory of n-person games, a theory that is considerably less sophisticated and more speculative than is that for two-person games. The zero- or constant-sum restriction is also bothersome, but, to some extent, this hurdle can be surmounted.*22

A Three-Person, Constant-Sum Game

As was the case with our model in the preceding chapter, it will be useful to “idealize” the institution under consideration, that is, to construct a model which will embody the essential characteristics of the institution without the complicating features. The model to be employed here must be even more restricted than the one used earlier. We shall initially assume that the total group is composed of three persons, equally situated. In order to relate the analysis to that of the preceding chapter, we may also assume that the individuals are farmers in a township interested in road repair. We shall assume further that the repair of one man’s road produces no external or spillover effects on other members of the group.

We assume that a decision has already been made to spend a total of $1 (additional zeros will not modify our analysis) on road repair in the whole township. For simplification, let us suppose also that this sum is not raised from general taxes but is instead received in the form of an earmarked grant from some higher-level governmental unit. This assumption assures us that the game we shall consider will be one of constant-sum at $1. We continue to assume that all decisions concerning the allocation of road-repair funds are to be made by simple majority vote, and that this is the only accepted way of making collective decisions. In our first model, we analyze the operation of this rule in an isolated, single action: that is to say, the $1 grant is received only once and it must be allocated once and for all and in complete abstraction from other collective issues that may arise.

This “game” may now be normalized and put in characteristic-function form as follows:

i.  v(1) = v(2) = v(3) = 0

ii.  v(1,2) = v(1,3) = v(2,3) = 1

iii.  v(1,2,3) = 1.

This characteristic function states the values of the various possible coalitions that may be formed. The function clearly shows that no “coalition” composed of less than two members of the group will have value, while all coalitions of two or more members will have a value of one. If the members of a winning two-person coalition choose to share their gains symmetrically, the following three imputations become possible “solutions”:

This set of imputations will be called F, or the F set. This set, and this set only, satisfies the Von Neumann-Morgenstern requirements for “solution” to n-person games, and may, in a restricted sense, be called the solution. The first of these requirements is that no single imputation in F either dominates or is dominated by any other imputation in the same set. (Domination is defined in terms of the effective decision-making subgroup or coalition: two in the model under analysis.) The second requirement is that any imputation not in F is dominated by at least one imputation in F.*23

The dominance aspects of the imputations in F may be illustrated with reference to proposed shifts to imputations not in F. Suppose that the imputation (0, ½, ½) is proposed by a majority coalition (2, 3). Individual 1 can propose an alternative imputation (¼, ¾, 0), which the coalition (1, 2) can carry (which dominates the first imputation). Individual 2 might be led to abandon the first coalition with 3 and support the modified proposal since his position will be improved (¾ > ½). However, this second imputation, which is not in F, will, in turn, be dominated by the imputation (½, 0, ½), which is in F for the majority (1, 3). Individual 2 may be wary about any initial departure from the coalition with 3 if he foresees the prospect of more than one move before action is finally taken.*24 Because of this fact, the imputations in F are presumed to be more stable than those not in F, although game theorists recognize and acknowledge the limitations on the ideas of “solution” and “stability” in the n-person game.

The set of imputations, F, contains the imputations that we could predict from the operation of majority voting in isolated actions. Two persons would tend to secure all of the benefits while the third person would secure nothing, assuming that each individual approaches the collective decision with a view toward maximizing his own expected utility, and assuming that individual utility functions are independent. Note that the set F includes imputations that dominate the “equitable” imputation (1/3, 1/3, 1/3).*25Any one of the three imputations in F dominates the equitable imputation with respect to a required number of individual voters. The equitable imputation would seem, therefore, to be the most “unstable” of all imputations since any majority can upset it. Compare this with another “weak” imputation not in F, say, (¼, ¾, 0). This imputation is dominated only by the imputation (½, 0, ½) in F, and by a limited subset of other nonstable imputations. Hence, to change from (¼, ¾, 0) to a solution in F, a particular majority (1, 3) is needed, whereas to shift from (1/3, 1/3, 1/3) to a solution in F, any majority will be sufficient. Thus, the “equitable” imputation may be stabilized only by significant departures by many individuals from utility maximization.

A Five-Person, Constant-Sum Game

Let us now extend this analysis to a five-person group, with the same initial conditions assumed. We continue to assume simple majority rule so that three persons are now sufficient for decision. The characteristic function is now as follows:

i.  v(1) = v(2) = v(3) = v(4) = v(5) = 0

ii.  v(1,2) = v(1,3) = . . . . . . . = v(4,5) = 0

iii.  v(1,2,3) = v (1,2,4) = v(1,2,5) = v(1,3,4) = v(1,3,5) = v(1,4,5) = v(2,3,4) = v(2,4,5) = v(3,4,5) = v(2,3,5) = 1

iv.  v(1,2,3,4) = v(1,2,3,5) = v(1,2,4,5) = v(1,3,4,5) = v(2,3,4,5) = 1

v.  v(1,2,3,4,5) = 1.

For the solution, set F, developed as before, we get:

Note that any one of these imputations in F dominates what we have called the equitable imputation (1/5, 1/5, 1/5, 1/5, 1/5) for the required decisive coalition of three persons. On the assumption of individual utility maximization, therefore, the equitable imputation would never be chosen.

It is clear that the analysis can be extended to a group of any size. The F-set, or “solution,” imputations will always contain only those involving the symmetric sharing of all gains among the members of the smallest effective coalition. In the game of simple majority rule the smallest effective set will approach 50 per cent of the total number of voters as the group is increased in size. Imputations within the solution set can always be found which will dominate, for an effective coalition, any imputation outside the set. As the size of the group is increased, however, the stability properties of the imputations in the set F seem to become less strong. In our earlier example of the three-person game, we found that the solution within the F set tends to be more stable than any similar set of imputations outside F because successful individuals might be able to foresee the consequences of departing initially from a coalition formed within F, which dictated that the gains be shared symmetrically among the members of the coalition. These consequences are, of course, that members of an apparently effective coalition might, before action is finally taken, be replaced by outsiders in a newly formed coalition.

It is perhaps useful to note that the argument for symmetry in the sharing of the gains among members of the dominant coalition rests on slightly different grounds than it does in the case with two-person co-operative games or in n-person games requiring that all participants must agree on a sharing arrangement. Schelling, in his recent argument for abandonment of symmetry, confined his discussion largely to these latter games.*26 If, as in the “majority-rule game” that we are considering here, the rules dictate that only a certain share of the total group need agree, the case for effective-coalition symmetry is stronger. The individual in the winning coalition will tend to be satisfied with a symmetrical share in total gains, not because he expects no member to concede him a larger share due to a general attitude of “fairness,” but because he knows that, if he does demand more, alternative individuals stand ready and willing to join new coalitions which could effectively remove his gains entirely.

As the total group grows in size, these effective restraints on individual action are weakened. The individual will reckon his own contribution to an effective coalition at a lower value, and he will be more tempted to depart from imputations within the “solution.” The outcome of the majority-rule game in large groups seems likely to be that predicted by our model of Chapter 10. Coalitions will be formed, but any single winning coalition will be relatively unstable and impermanent. On the other hand, it should also be emphasized that as the size of the group becomes larger, any tacit adherence to moral or ethical restraints against individual utility-maximizing behavior also becomes much more difficult to secure. The deliberate exploitation of the third member by any two members of a three-man social group may be difficult to conceive, but the individual’s interest in his fellow man falls off quite sharply as the group is enlarged. In this sense, therefore, the basic assumptions of the game-theory model become more relevant for large groups than for small ones. The concept of “solution” may be considerably more fuzzy in large-group situations, but the direction of effect that may be predicted to emerge seems to be of significant relevance for any study of real-world political decision-making.

The Limitation of Side Payments

We have analyzed the operation of majority voting in the simplest of models. We have assumed the group to be confronted with a single issue that was to be decided once and for all. As applied to real-world institutions, the limitations of this model must be carefully kept in mind. Many of these have been obscured in the analysis above, and some of them must now be mentioned. In the first place, as we have suggested in Chapter 10, logrolling or vote-trading processes would tend to arise when more than a single issue is presented to voters. We propose, however, to leave this complication aside for the time, and to assume that all forms of vote-trading are prohibited in some way. If we want to employ the terminology of game theory here, we may say that all side payments are prohibited. This prohibition effectively prevents the individual voter from being able to express his intensity of preference for or against the specific measure proposed. All that he may register is the direction of this preference, not the intensity. Implicit in the support of decision-making institutions and rules which do serve, wholly or in part, to limit side payments seems to be the psychological assumption that individual preferences are essentially symmetrical.*27

Let us see precisely what this complete prohibition of all side payments implies for our “solution” imputations. Consider the same three-person game discussed above, in which the $1 grant is to be divided among the three roads, with each repair project benefiting only one individual. Let us assume that, in actuality, road repair is highly productive on only one of the three roads, moderately productive on the second, and not worth the cost on the third. The values resulting from one-half (50¢) of the total expenditures on each road, respectively, are as follows: $1, 50¢, 25¢, or to use fractions: 1, ½, ¼ (note that these are not imputations). Simple majority voting, with all side payments (open and concealed) being prohibited, will convert all such “political games” into a fully normalized form. The solution set of imputations will be the same as before. Quantified or measured in terms of input or cost values, this set is:

It is now necessary, however, to distinguish between input or cost values and output or benefit (utility) values. The latter become, in the same set of imputations:

The important conclusion here is obvious. In benefit or productivity terms, the “game” is not constant-sum, and, with all side payments prohibited, there is no assurance that collective action will be taken in the most productive way. There is no more likelihood that the first imputation will be chosen than the second or third. The rule is as likely to select the least “productive” imputation as it is the most “productive.”*28

The prohibition of all side payments also prevents any imputation being selected which directly benefits less than a simple majority of the voting population, regardless of the relative productivities of public investment. For example, let us now suppose that the $1 grant, if expended exclusively on the first road, would yield a benefit value of $10, on the second road $5, and on the third road only $1. If, in fact, all funds were expended on the first road, the imputation would be (10, 0, 0). However, note that any imputation such as (0, 2½, ½) would dominate the more concentrated, but more productive, investment. The set of imputations having the solution properties under the conditions outlined would be:

These rudimentary elements of game theory have helped us to demonstrate in a somewhat different, and perhaps more decisive, manner the effects of simple majority rule that were already discussed in Chapter 10. If some vote-trading is not introduced, no allowance can be made for possible variations in individual intensities of preference, a point that is rather dramatically shown in a quantitative way in the last simple model.

Allowance of Side Payments

The apparent distortions that may be produced by the operation of simple majority rule without side payments suggest that the model with side payments be examined. Side payments may “improve” the results. We propose, therefore, to examine this prospect more carefully. Let us now suppose that there exists complete freedom for individuals to make all of the side payments or compensations that they choose to make. No restrictions are placed on the methods of payments, but we may think of them as being made in generalized purchasing power, or money. Such behavior of individuals is assumed not to be prohibited by either legal or moral restraints. This model allows us to introduce something akin to vote-trading in the model without departing from the confines of a single, simple issue.

Let us assume the existence of the last benefit schedule mentioned above: that is, if the whole grant were to be expended on each road, the “productivities” would be, respectively, $10, $5, and $1. Simple majority voting, with full side payments, will now produce a “solution” set of imputations as follows:

In the first imputation, Individual 1 gets all of the grant expended on the repair of his own road, but he must pay Individual 2 one-half of the monetary value of the net gains for his political support. In the second imputation, Individuals 2 and 3 simply trade places. The third imputation in the solution set is most interesting. Here all road repairs are still carried out on the first road, where investment is far more productive than on the other roads, but Individuals 2 and 3 form the political majority which forces Individual 1 to pay full compensation for the road repair that he secures. Despite the fact that only his road is repaired, Individual 1 is no better off after collective action is taken than he is before.*29

We see that the results of simple majority voting in the model where full side payments are allowed differ in several essential respects from the results of this rule when such payments are not allowed. First of all, side payments insure that the funds will be invested in the most productive manner. Secondly, there is no requirement that the projects undertaken provide physical services to more than a majority of the voters. As in all of the earlier models, the solution will embody a symmetrical sharing of total gains among the members of the smallest effective coalition, but note that the introduction of side payments tends to insure a symmetric sharing of gains measured in benefit or productivity terms.

In contrast to a logrolling model, the model which does include open buying and selling of votes (that is, full side payments in money) does not seem characteristic of modern democratic governments. We do not want to prejudge the ethical issues introduced by this model at this time, but commonly accepted attitudes and standards of behavior, as well as established legal institutions, prevent any approach to full side payments being carried out in actuality. In spite of this, the model is a highly useful one in that it does point to the type of solution attained under the more complex models which allow indirect side payments to be made.

Simple Logrolling and Game Theory

We refer, of course, to those vote-trading or logrolling models that have been discussed in Chapter 10. The simple logrolling model falls halfway between that containing no side payments and that which allows full side payments. In order to introduce logrolling, we must depart from single issues and assume that the group confronts a continuing series of separate measures. In game-theory terms, logrolling is simply an indirect means of making side payments. Individuals are unable to “purchase” voter support directly with money, but they are able to exchange votes on separate issues.

Let us continue to employ the road-repair example, with the prospect of a $1 grant from external sources being made available to the community for disposition in each of a successive number of time periods. Let us also assume the same payoffs as before: namely, that the productivity of a $1 investment on Road 1 is $10, and on Road 2, $5, and on Road 3, $1. We must also now make some assumption about the marginal productivity functions in this model. We shall assume that, over the range of decisions considered in any bargain, the marginal productivity of investment on each road is constant: that is to say, the productivity of any $1 investment on Road 1 is $10, regardless of the amount of incremental investment undertaken on that road in previous periods.

Recall that under simple majority voting without side payments the solution set of imputations, measured in benefit terms, was:

while in the model with full payments, this set was:

In the first case, the repairs would be carried out on any two of the roads represented in an effective coalition, not necessarily those roads most in need of repair. In the second case, the repairs would tend to be made where the investment is most productive, with a side payment or payments being made to insure sufficient support in the voting process.

In our simple logrolling model, the only way in which the first individual can “purchase” support for repairs on his road is by agreeing to vote for the repair of some road other than his own. He cannot substitute for this the more “efficient” transfer of money. It is difficult to present the results here in terms of a single set of benefit imputations because we must include a whole series of issues, but clearly these results must approach more closely those of the first rather than those of the second alternative model. Since some funds must be devoted to relatively unproductive investment, in some periods, the greater “efficiency” of the second model cannot be secured. We may convert simple logrolling into a political game by considering a single road-repair project in which the individual beneficiary secures majority support by giving promises of reciprocal support on future proposals, with these “promises” commanding some current economic value. The general logrolling model can then be thought of as consisting of a sequence of such games. There are, however, some differences between the simple logrolling model or its game analogue and the basic games discussed earlier. Simple logrolling, even if the issues are closely related to each other, can introduce minimal improvements in “efficiency.” The process removes the necessity of insuring some physical benefits to an absolute majority for each single piece of legislation. Road repairs could, in any one period, be devoted exclusively to one road. Moreover, if there should exist important returns to scale of single-period investment, this could produce significant efficiencies.

Our general logrolling model can best be interpreted on the assumption that the political process embodies a continuing series of issues: in specific reference to the illustration, separate road-repair proposals. If, however, all road-repair projects must be voted on a single omnibus proposal, the results become equivalent to those demonstrated in the elementary games previously discussed. In this case, a minority of farmers will secure no road repairs, whereas in the general logrolling model, even under majority rule, each road would tend to be repaired because of the multiplicity of issues allowing for many separate coalitions. This difference between these two majority-rule models, however, will not affect the individual constitutional evaluation of majority voting as a means of making political decisions. In the one case, external costs will be expected because of the excessive road repairs generally carried out; in the other, external costs will be expected because of the fact that the individual might occasionally find himself in the losing coalition on a single, large, omnibus issue.

Complex Logrolling

In our example, we have discussed the game theory aspects of logrolling phenomena that are confined to closely related issues. Instead of this, logrolling may actually take place by the trading of votes over a wide range of collective decisions, which may or may not bear physical resemblance to each other. As the “bargains” expand to include more heterogeneous issues, it seems clear that the results will begin to approach those emerging from the model which allows unrestricted side payments. If there is a sufficient number of issues confronted by voters at all times, and if the range and distribution of the individual intensities of preference over these issues are sufficiently broad, the complex logrolling process may approximate unrestricted side payments in results. Insofar as this is true, the full extent of the differential benefits from public outlay, or the differential costs of general-benefit legislation (that is, the differential intensities of individual preferences), can be exploited. The individual voter who is either strongly opposed to or strongly in favor of certain measures may, if necessary, “sell” his vote on a sufficient number of other issues to insure victory for his side in the strongly preferred outcome. His “purchasing power” is determined by the value of his support on all issues considered by other voters. Of course, the individual voter will rarely want to use up all of his purchasing power on any single measure, just as the individual consumer in the marketplace rarely uses up all his purchasing power on a single commodity or service. Complex logrolling of this type remains a “barter” system, but it merges into a pure “monetary” system (that is, one with full side payments) as the range of issues undertaken collectively is broadened. Implicit logrolling (discussed in Chapter 10), in which the voter is presented with a complex set of issues at the same time, is one form of the complex logrolling discussed here. If the voter is enabled to choose from among a sufficiently large number of alternative sets, his effective “purchasing power” approaches the limit that would be available to him under a “monetary” system.

The “Individual Rationality” Condition

To this point our models have been simplified by the assumption that the choice or choices facing the group involve only the final sharing of an earmarked grant or grants received from external sources. We now propose to make the models somewhat more realistic by dropping the external-grant features. Let us now suppose (just as we did in Chapter 10) that all funds for road repair are to be raised from general taxes levied uniformly on all citizens. We return to the simplest three-person game initially analyzed. This “new” game can also be discussed in the normalized form. To do so requires only that we attribute a fixed monetary sum to the various individuals at the outset. In the three-person game let us suppose that each person retains, at the beginning of “play,” $1/3; the beginning imputation is (1/3, 1/3, 1/3). Now assume further that “play” is to involve, in every case, the disposition of $1. The form of the characteristic function is not changed:

i.  v(1) = v(2) = v(3) = 0

ii.  v(1,2) = v(1,3) = v(2,3) = 1

iii.  v(1,2,3) = 1.

As in the earlier game, the individuals acting jointly as a group, [v(1, 2, 3) = 1], for example, under a rule of unanimity, cannot receive more than the gainers receive from the formation of coalitions under simple majority rule. There is, however, one major difference between the game now under consideration and the simpler one discussed earlier. In the previous game there could exist complete individual freedom to withdraw from the group. Since the funds to be expended there were assumed to come from outside the group itself, the withdrawal of a member would not serve to reduce the total gains to be secured. In other words, the earlier game satisfied a condition which may be represented as an adaptation of what Luce and Raiffa call the condition of individual rationality.*30 They define this condition as follows:

v({i}) 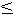x_i for every I in I_n.

This condition states that no individual in the whole group, I_n, will ever receive less by being in the “game,” regardless of whether or not he is in the winning or losing coalition, than he would if he “played alone” against all other members of the group. Applied to our particular problem, “playing alone” ({i}) may be interpreted as withdrawal from the game altogether.

The relevance of this condition is obvious when the purpose is that of analyzing “voluntary” games, and when it is further recognized that most of the game situations in which the individual finds himself do, in fact, represent such voluntary games. The extension of game-theory models to any analysis of political decision-making requires some consideration of “coercive” games. The condition of individual rationality, as we have stated it above, need not be satisfied at all. The individual participant in collective decision-making may, in many of the actual choices made through the political process, prefer to withdraw from “play.” This does not suggest that the individual necessarily would want to withdraw from participation in the whole set of games represented by state action (although, conceptually, he could also want to do this). In any case, the individual can normally neither choose the political “games” in which he desires to participate nor can he withdraw from the ultimate social contract readily. He must remain as a participant on each issue that the group confronts.

Returning to the simple game before us, the individual, if he should be allowed to withdraw, could always retain his original value of $1/3. It follows that he would not voluntarily accept an expected value of less than 1/3 in any game if he were offered the alternative of not playing. However, in political groups, such action is not normally possible. Individuals cannot refuse to pay taxes even though they find themselves in a minority.

The solution set of imputations, in cost values, will be equivalent to that in the initial three-person game:

In each of these imputations, one of the three persons will be made worse off than when play begins. However, as a member of the political unit for whom decisions are being made, he is forced to submit to the results indicated by the operation of the rules.

The Limits to “Social” Waste

The majority-rule game considered here results in a net transfer of real income from one member of the three-person group to the other two members. Such transfers could, of course, take place directly without any necessity that tax revenues be expended in the provision of public services. In constitutional democracies, however, some limitations on majority action are almost always to be found. Moreover, since the individuals in our model are assumed equal in fiscal capacity at the outset, directly redistributive transfers would probably be prevented by constitutional provisions and traditions. If such transfers are prohibited, the majority coalition may effectively exploit the minority only through levying general taxes to provide special benefits, or through financing general benefits by special taxes. With this in mind, we shall now consider the extent to which the operation of simple majority voting rules can produce “social” wastage of resources.

If the solution set of imputations shown above is assumed to represent the imputed sets of individual evaluations of the public services (road repairs), note that there is no over-all wastage of resources. No “inefficiency” is introduced by the combined taxing-spending operation. The imputation (½, ½, 0), for example, means, in this sense, that an expenditure of $½ on the first person’s road yields to him an estimated value of $½; similarly, for the second man. The total additions to utility created by the expenditure of the $1 are valued at the same total as are the total subtractions from utility caused by the necessary taxes (½ + ½ = 1/3 + 1/3 + 1/3). The “productivity” of the public expenditure is exactly equal to the alternative “productivity” of the resources should they have been left available for private disposition. This means that no introduction of side payments could modify the results, which are identical to those of purely redistributive transfers. Such transfers, by definition, involve no “social wastage” in the sense considered here, assuming, of course, that the supplies of the productive factors are not affected.

Let us now suppose, however, that the expenditure of $½ on the first person’s road yields to him an incremental utility that he values at $5/12, and similarly for the second and third man. Under this modified assumption about the productivity of road repairs, we get a set of possible solution imputations as follows:

Note that it will still be profitable for the members of the winning coalition to play the game (5/12 > 1/3), but the total estimated value of the “gains” is less than the “losses” (10/12 < 1), or, in net terms, (1/3 > 1/6). If these individual evaluations can be compared in some way, then clearly “social wastage” of resources must be involved in the carrying out of the majority decision. One means of allowing some comparison of individual utilities is, of course, that of allowing side payments. If these are introduced, the set of imputations above cannot be said to represent any solution. Instead, in each imputation the person in the minority could always offer to compensate at least one of the others in order to get him to refrain from playing. For example, the imputation (11/24, 11/24, 2/24) outside the set above is dominated by no imputation in the set. Hence, the set of possible solution imputations,

does not satisfy the Von Neumann-Morgenstern requirements. In this situation it does not seem likely that the “game,” which must be negative-sum, will be played at all. No road repairs will be undertaken.

It should be remarked, however, that this result follows only if side payments are allowed. If neither purely redistributive income transfers nor side payments are possible, there is nothing that can arise to prevent the social process from proceeding, even if, translated into game-theory concepts, the game is one of negative-sum. Under the same productivity assumptions as before, the set of imputations,

now takes on all of the characteristics of the Von Neumann-Morgenstern “solution.” The person in a minority position can offer a maximum of 1/3 to another to refrain from playing.

It is reasonably clear from this analysis that the limits to resource wastage that could possibly result from the operation of simple majority rule will be determined by the size of the group. In our model three-person group, a “total productivity” of public investment must be at least two-thirds as great as that sacrificed in the private sector. In a five-person group this fraction becomes three-fifths. The maximum limits to resource wastage are defined by the fraction M/N, where M is the minimum number of voters required to carry a decision, and N is the number of voters in the whole group for which choices are to be made. Thus, at the limit, a public-investment project need only be slightly more than one-half as productive as the private-investment projects that are sacrificed, productivity in each case being measured in terms of the individual evaluation of benefits.*31

This analysis is not intended to suggest that majority-rule “games” will tend to be constant- or negative-sum. In many cases, the game will, of course, be positive-sum. By altering the productivity assumptions of our simple models here, the results of positive-sum games are readily attainable. Let us suppose that the investment of $½ on each road yields $1 in benefits, as estimated by the individuals themselves. The “solution” set of imputations becomes:

Note that here, as in the constant-sum case, the introduction of side payments will not change this solution. Under the conditions outlined, the introduction of side payments will change the solution only if the game is negative-sum.

This limitation is no longer present, however, if we introduce some asymmetry in the benefit schedules, that is, if we assume that the productivity of public investment may vary from road to road in our model. We can, of course, conceive of games with asymmetrical benefit schedules which are positive-, constant-, or negative-sum. Moreover, a game may be switched from positive- to negative-sum within a single “solution” imputation. Consider the following set:

Let the imputed values represent the estimated individual evaluations of the public investment of $½ on each road. Thus, the set takes on the properties of a solution unless side payments are allowed to take place. No imputation in the set is more likely to be chosen than another. If the first imputation is chosen, the game, for the whole group considered as a unit, is positive-sum (17/12 > 1); if the second imputation is chosen, constant-sum (1 = 1); if the third imputation, negative-sum (7/12 < 1).

The introduction of side payments will insure that the second and the third imputations would never be produced, and even the first imputation would not exhibit the required stability properties required for solution. The F set would in this case become

assuming constant returns to investment on the first road.

The General Benefit-Special Taxation Model

The previous models have incorporated the assumption that public projects providing differential benefits to individual citizens are financed by general taxes imposed equally on all citizens. The elementary propositions of n-person game theory applied to these models enable us to predict that serious resource wastage can result from the operation of simple majority rule. The reasons are the same as those discussed in Chapter 10. Majority rule allows members of the decisive coalition to impose external costs on other individuals in the group, costs that are not adequately taken into account in the effective decisions. Aggregate marginal costs exceed the aggregate marginal benefits from public investment. Relatively too many resources are invested in the type of public projects analyzed in the model—relatively too many as compared with both alternative private employments of resources and with alternative public employments.

The assumption that general taxation is levied to finance special benefits is clearly more descriptive of real-world fiscal institutions than the converse case. Ethically accepted principles which have long been espoused and which have found expression in modern tax institutions stress the importance of generality in the distribution of the tax burden among members of the social group. No such principles have guided the distribution of public expenditure among the several possible uses. However, in order to make our analytical models complete, it will be useful to modify our assumptions and to consider the reverse situation. Let us try to apply the elementary game-theory constructions used above to the model in which collective goods, providing general (equal) benefits to all citizens, are financed by discriminatory taxation. The analysis is relatively straightforward, but, interestingly enough, this model is not symmetrical in all respects with the one previously considered, as we shall demonstrate.

We begin, as before, with an initial imputation (1/3, 1/3, 1/3), which represents asset values held by the individuals. We now introduce a general-benefit situation. Suppose that the group is confronted with the opportunity to purchase a genuinely collective good, the benefits from which are not divisible; if one individual secures these benefits, each individual in the group must secure them in like amounts. As a first example, let us assume that each individual estimates his own benefits from the good to be 1/12. Assume further that the total costs of the collective good are 4/12 or 1/3. If the good is purchased, the final imputation of benefits, from the collective good alone, must be (1/12, 1/12, 1/12). However, what is relevant in this case is the “net” imputation that will result from the purchase of the collective good and the retention of shares of the initial assets.*32 The effective coalition will tend to impose special taxes on the minority, producing a “solution set” as follows,

assuming that side payments are not allowed. The over-all investment is not worth the cost (3/12 < 4/12); but, if taxes can be imposed in a discriminatory manner, it will still be an advantageous project from the point of view of the members of the effective coalition (5/12 > 4/12). The game in our illustrative example is negative-sum. Positive- or constant-sum games can also be constructed in this framework. Our purpose in this illustration is to demonstrate the possibility of negative-sum games being played and, thus, the possible wastage of resources. In the illustrative example here, the public investment should not have been undertaken since the resources employed are more productive if left in the private sector of the economy.

It can readily be seen that there are no effective limits to the possible extent of resource wastage under the assumptions of this model. Any project yielding general benefits, quite independently of cost considerations, will be supported by the dominating majority if they are successful in imposing the full tax financing of the project onto the shoulders of the minority. This feature differs substantially from the general-taxation model, where some quantitative limits could be estimated for the degree of resource wastage made possible under majority rule. Note that this feature also differs from the general implications of the logrolling analysis of Chapter 10. The analysis there implies that general-benefit projects would tend to be slighted in favor of special-benefit projects. This implication must be carefully constrained; it remains clearly true only if the assumption of general taxation is retained. If discriminatory taxation is allowed, there seems to be no a priori reason to expect special-benefit projects to take a dominating role in the operation of majority rule, except for the general presupposition that individuals may be more interested in special-benefit projects.

There is another important respect in which the general-benefit model is asymmetrical with the general-taxation model. In the latter, we have been able to demonstrate that, under the operation of simple majority rule, relatively too many resources are likely to be devoted to special-purpose public-investment projects. To be fully symmetrical with this, the general-benefit model might appear to require the conclusion that relatively too few resources be devoted to general-purpose public projects. This conclusion, however, cannot be supported. It can be demonstrated that relatively too many resources will be devoted to both special-benefit and general-benefit public projects under the operation of simple majority rule. This is an especially significant implication that emerges from our application of game theory to this voting rule, and the demonstration deserves to be carefully presented.

We shall show that every general-benefit project that is worth its cost will tend to be adopted by simple majority voting: that is to say, we shall try to prove that all possible projects involving resource investment more “productive” than the alternative investment in the private sector of the economy will tend to be adopted by majority rule. If this can be demonstrated to be true, our main point will have been established because, in the illustrative model first employed in this section, we have shown that some unproductive projects (negative-sum games) will be selected.

The proof is almost intuitive. If the dominant majority is able to impose the full costs of general-benefit projects on the minority, it follows that all projects yielding any benefits at all to the majority coalition members, and costing no more than the maximum taxable capacity of the minority, will be adopted without question. In our current example, any general-benefit project (any pure collective good) that costs up to 1/3 will surely be adopted. This is because, if discriminatory taxation is allowed, a sum up to this amount may be collected from the single minority member of the group. Hence, for all such projects a member of the majority coalition may secure some net benefit without cost to himself.

As the costs of collective goods move beyond the maximum taxable capacity of the minority member of the group, beyond 1/3 in our example, the individual members of the majority will be able to balance off gains against costs. Since they are the residual taxpayers, their own calculus will insure that a more than satisfactory balancing off will be achieved. Any project will be adopted that provides the group with general benefits valued more highly than the alternative private investments. While it is true that in making their final decisions they do not include in their calculus the full marginal benefits of the collective goods, because, by definition, these goods provide benefits to all members of the group equally, neither do the members of the majority include the full marginal costs. Moreover, the calculus will always reflect a more accurate estimate of marginal benefits (since the minority members will receive only an equal share) than of marginal costs (of which the minority members will bear more than an equal share).

In our analysis of the general-benefit model, we have not introduced side payments. If these are introduced, the effects are similar to those traced in the general-taxation models. Side payments will insure that no negative-sum games will ever be played: that is to say, “unproductive” public investments could never be undertaken if full side payments were to be permitted. If indirect side payments in the form of logrolling are allowed, some mitigation of the resource wastage involved in the operation of majority rule decision-making is to be expected. The extent of this mitigation will be dependent on the extent and range of the logrolling that takes place.

The General Taxation-General Benefit Model

Many of the modern activities of governments can be classified as falling within one of the two models previously discussed or in some combination of the two. For completeness, however, there remains the examination of those activities undertaken by governments that provide general benefits and are financed from general taxation. Let us assume that a community of identical individuals is faced with the task of providing a genuinely collective good. Benefits from this good are to be distributed equally among all citizens. This good is to be financed by taxation that is also equally distributed among all citizens.

It is immediately clear in this model that the collective-choice process does not take on the attributes of a game, regardless of the rules that may be adopted for decision-making. In this model the political process offers to the individual participant no opportunity to gain differential advantage at the expense of fellow participants. When the individual makes a decision, under any rule, he must try to compare the advantages that he will secure from the availability of the collective good and the costs that he will undergo from the increase in the general tax. His behavior can exert no external effect, either in costs or benefits, on third parties.

Communities are not, of course, made up of identical individuals. Moreover, once differences among individuals in tastes, capacities, endowments, etc., are admitted, the model for general taxation and for general benefits becomes much more difficult to discuss. It remains possible to imagine a collective decision in which the benefits from the public services provided are distributed among the membership of the group in such a manner as to precisely offset the distribution of the tax burden for this particular extension of service. In this case, where public expenditure is financed solely on some principle of marginal-benefit taxation, the conclusions reached above will hold. The individual cannot benefit at the expense of his fellows through the political process, and the game analogy breaks down. It is clear, however, that this model cannot be observed in the real world. We know that public services provided by governmental units do exert differential benefits and that these services are financed by taxation that is not general in the sense required for this extreme conceptual model.

The usefulness of this model lies in its implication that, insofar as collective action takes on such characteristics of generality (that is, nondiscrimination), the applicability of the game-theory conclusions is reduced. As we have emphasized elsewhere, the trend away from general legislation toward special legislation in modern democracies makes the conclusions drawn from the game-theory analogues more applicable than they might have been a century past.

Conclusions

The generalized conclusion that may be reached as a result of the application of elementary game theory to the institution of simple majority voting is evident. There is nothing inherent in the operation of this voting rule that will produce “desirable” collective decisions, considered in terms of individuals’ own evaluations of possible social alternatives. Instead, majority voting will, under the assumptions about individual behavior postulated, tend to result in an overinvestment in the public sector when the investment projects provide differential benefits or are financed from differential taxation. There is nothing in the operation of majority rule to insure that public investment is more “productive” than alternative employments of resources, that is, nothing that insures that the games be positive-sum. Insofar as the vote-trading processes which emerge out of the sequence of separate issues confronted produce something akin to side payments, this resource-wasteful aspect of majority voting will tend to be reduced in significance.

The whole question of the relationship between the operation of simple majority voting rules and the “efficiency” in resource usage, within the context of the game-theory models, can best be discussed in terms of the constructions of modern welfare economics. In the following chapter we shall introduce these constructions in specific reference to the analysis of this chapter.