The Calculus of Consent: Logical Foundations of Constitutional Democracy

Our analysis of direct democracy can, we think, be extended to almost any set of political institutions while still retaining much of its explanatory and predictive value. We shall discuss this extension in Chapter 15, but now we shall proceed to analyze the operation of decision-making rules in terms of simple models involving individual participation in collective choices at the operational level. We shall occasionally refer to the action of legislative assemblies which seems to conform to the implications of our analysis. In one sense, these phenomena confirm the hypothesis that our model is of general relevance.*1

The Time Sequence of Collective Decisions

Perhaps the most distinctive feature of our models, in comparison with other analyses of collective choice-making, is the central place assigned to the plurality of collective decisions over time. The analysis is not designed to explain the operation of decision-making rules on single, isolated issues. The analytical problem posed is that of examining comparative rules for choice as these apply to many decisions spread over “time.” Any rule must be analyzed in terms of the results it will produce, not on a single issue, but on the whole set of issues extending over a period of conceptually finite length.

The individual participant’s recognition that issues for collective choice are not unique and isolated events imposes severe limitations on any analysis of single decisions. Issues may be wholly unrelated in their descriptive characteristics, but the rational participant will recognize the time sequence of political choice. Moreover, this will cause him to seek “gains from trade,” when possible, by exchanging his vote on one issue for reciprocal support of his own interest by other participants on other issues. Thus, the time sequence of collective choice is very important in that it allows us to introduce an economic dimension to individual votes somewhat more handily than would otherwise be the case.

The difficulty of attributing such an economic dimension to votes in the political process has long been one of the stumbling blocks in the extension of economic reasoning to political models. The economic value of votes is confirmed by the selling and buying activities of individuals in “corrupt” circumstances, but models based on this “immoral” behavior pattern have not been considered to be useful in analyzing accepted political behavior. In the latter the essential requirement of scarcity has not been incorporated in the models, with the result that the applicability of an economic approach has been sharply limited. The individual participant normally has a single vote on each separate issue; votes do not “run out” or get “used up” as do the allegedly analogous “dollar votes” of individual participants in market choices. There seems to have been present a rather common failure to recognize the simple fact that if political votes did not have economic value, “corruption” would be impossible.

Individual votes result in collective decisions that exert economic effects. Each decision can be described in terms of its effects on individual incomes and wealth. So defined or described, the collective decision assumes a time dimension; it can be located in time and its impact can be measured over time. The political vote that assumes economic value can only refer to the vote exercised when decisive action is taken. The opportunity for the decision-making group to modify and change a provisionally approved decision through various forms of repeat voting represents yet another factor that has caused the application of an economic dimension to the political vote to be neglected.

Individuals’ votes have economic value. Moreover, for any commodity or service having economic value, a market will tend to emerge from the ordinary self-seeking behavior of men unless there are strong legal or moral prohibitions against trade. Such prohibitions are, of course, present to prevent the development of open markets in individual votes,*2 but this does nothing toward removing the economic content. The absence of open markets serves only to prevent the full utilization of the pricing mechanism in allocating the scarce elements among competing alternative uses. Moreover, if pricing cannot be employed, some substitute means of rationing must be introduced. There are an almost infinite number of schemes that could be devised, and each scheme can be described by a set of voting rules. In each case valuable individual votes will be distributed on some basis, and this basis may be wholly unrelated to individual evaluations.

Let us look briefly at an example. Suppose that the group is required to make only one collective decision. It must decide how to divide up the one and only lot of manna that has fallen from heaven. There are five members of the group, and the constitution dictates that all collective decisions are to be made by simple majority rule. This means that three, any three, of the five members must agree. Since buying and selling votes is ruled out, and since there is only one decision to be made, the first three individuals who form a voting coalition will secure the manna. The two in the minority may place a much higher value on the manna than any one of the three winners, but this is irrelevant to the decision. We shall discuss models similar to this one in much greater detail later. Our purpose here is to indicate not only that any voting rule acts as a means of rationing, but that this rationing may cause a distribution of collective “goods” that is wholly unrelated to individual evaluations.

We note, however, that the introduction of a time sequence of political choices allows a market of sorts to be developed without the necessity of changing the rules for decision on single issues. If the individual participant recognizes the economic value of his own vote to others on certain issues and, in turn, recognizes the economic value of others’ votes to him on separate issues, he will be motivated to engage in “trade.” Moreover, if ways of “trading” can be found that do not clearly conflict with accepted standards of behavior, individuals will seek mutual advantages in this way. The possibility of exchanging votes on separate issues opens up such trading prospects. The individual may effectively, but imperfectly, “sell” his vote on a particular issue, securing in return the votes of other individuals on issues of more direct interest. This process of “logrolling” will be carefully analyzed in the following chapter, but some preliminary points should be made here.

With relatively few exceptions logrolling phenomena have been viewed as deviations from the orderly working of the democratic process. This view seems to have been adopted for two separate reasons. First, and more important, the economic motivation for political behavior reveals itself most clearly in the occasional examples of Congressional logrolling legislation. Students of the political process, who adopt the view that, at base, political behavior is not motivated by economic interest, must explain such action in terms of aberrations from more orthodox behavior. Secondly, and related to the first, there has been a failure to recognize that logrolling phenomena are much more pervasive than the more obvious examples would indicate. The phenomena surely occur at several levels of political sophistication, and the fact that the cruder instances occur at all should give the student of political process cause for looking somewhat carefully for more “acceptable” means of accomplishing similar purposes.*3

It seems clear that, insofar as divergent interests affect the political choices of individuals and groups, the logrolling process provides the general model for analyzing the various choice-making rules.*4 Surely the individual participant in collective choice recognizes the time sequence of events requiring collective action, and, just as surely, he will be motivated to engage in mutually advantageous “trades” or “compromises” with his fellows. The cruder models, in which the trade is made explicit, are useful in that they are more readily subject to analysis, but the more important cases probably occur beneath the outwardly visible surface of “politics.” The assumption that these crude models provide a general approach to the operation of political rules seems considerably more acceptable than the contrary one which assumes that the analysis of rules on the basis of single issues is a more satisfactory approach to a general theory of collective choice.

Perfect and Imperfect Markets

When a time sequence of issues is allowed for, some trading of votes takes place. No longer does the decision-making rule alone serve as the rationing device. An illustrative analogy may be helpful. Suppose that all rents on dwelling accommodations are strictly controlled, and at levels much below hypothetical “market” values. Individual landlords are subject to prosecution if they accept direct money payments (“bribes”) above the controlled rents from prospective tenants. On the other hand, they are not prevented from entering into other “exchanges” with tenants at freely determined and mutually advantageous terms of trade. Landlords may “sell” furniture to tenants, or they may “purchase” other commodities. Under circumstances such as these, the expected results would be less arbitrary than under the alternative system in which no free “exchanges” between landlord and tenant are allowed, that is, in which housing is rationed solely on a nonprice basis. On the other hand, the nonprice aspects of the “market” system would make the expected results diverge significantly from that which could be predicted to emerge from a completely free market in rental units.

In our rent-control analogy, to which we shall return in a later chapter, the combination of price and nonprice rationing appears as a special institutional pattern. In the political-vote case, however, this in-between or “imperfect” model represents, perhaps, the most general model of democratic process. This “imperfection,” however, makes the analysis especially difficult.*5 For this reason we shall find it necessary, in the chapters that follow, to employ extremely simplified models.

Some predictions concerning the results to be expected from the operations of the in-between model may also be derived by considering the alternative models that bracket the logrolling or imperfect-vote marketing model. As we have suggested, other scholars have analyzed the nonprice model, being forced to do so by their concentration on single issues. To our knowledge, however, the full price-rationing model has not been fully developed: that is, the model in which political votes are freely marketed for money has not been subjected to rigorous analysis, even for simple voting rules. The tools supplied by modern game theory are helpful in this respect, and in Chapters 11 and 12 we analyze the operation of simple majority-rule games under the assumption of full side payments. By relaxing the full side-payments assumption, we may also compare this model with one more closely approximating the logrolling model.

The Intensity of Individual Preference

Much of the traditional discussion about the operation of voting rules seems to have been based on the implicit assumption that the positive and negative preferences of voters for and against alternatives of collective choice are of approximately equal intensities. Only on an assumption such as this can the failure to introduce a more careful analysis of vote-trading through logrolling be explained. If all intensities of preference are identical over all individuals and over all issues, no trading of votes is possible. In this case the individual feels as strongly on one issue as on any other, and he will never rationally agree to exchange his vote for reciprocal favors.

An example may be helpful. Consider a society confronted with three issues in sequence. The group must choose between A and 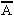, between B and 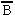, and between C and 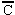. Let us assume that the constitution dictates that each of these issues shall be decided by simple majority voting rules. Assume that, in each case, 51 per cent of the voters favor the first alternative and 49 per cent favor the second alternative, but assume also that the majorities and the minorities are not uniformly composed over the three issues. If all preferences are equal in intensity, no bargains can be struck, and A, B, and C will be chosen. Consider Voter I who favors A, B, and 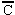, and Voter II who favor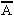s , , and C. Neither would be willing to trade his vote on two issues for the other’s vote on one issue, and a one-for-one trade would not be mutually advantageous.

Intuitively the assumption of equal intensity of preference seems unacceptable. Clearly the more general assumption is that individual “tastes” for collectively obtained “goods” vary in both object and intensity. In the extremes there would seem to be no question of such variance. If the issue to be decided is whether or not Voter I will or will not be executed, the intensity of preference of Voter I against this action will clearly, in some circumstances, be greater than the desires of other voters in favor of the action. As with certain other aspects of political theory, there seems to have been a failure here to distinguish between positive analysis and normative theory. Implicit in much of the discussion of majority rule has been the idea that individual votes should be treated as reflecting equal intensities of preference, quite independently of whether or not the norms agree with the facts in the case. This idea, in turn, probably stems from the more fundamental norm of democratic organization—that of political equality. Political equality may be fully accepted as essential to any form of democratic process, but this does not imply that individual votes on particular issues should be considered as if they reflect equal intensities of preferences over all participants.

The assumption of equal intensity of preference for all voters over all issues really amounts to imputing to each individual a most restricted utility function, and one that is wholly different from that which is employed in economic analysis. Not only is utility measurable; it is directly comparable among separate individuals. To the modern economist this approach to individual calculus seems anachronistic and sterile.

Equal Intensities and Majority Rule

Although we do not propose to discuss the equal-intensity assumption in detail here, a brief digression on the relationship between it and simple majority rule may be worthwhile. When all individual preferences are of assumed equal intensity, simple majority rule will insure that the summed “benefits” from action will exceed the summed “losses.” In this way simple majority rule appears to assume a unique position in terms of a very restricted “welfare” criterion.

Consider our earlier example. Recall that 51 per cent of the voters favor A and that 49 per cent favor 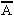, and that positive and negative intensities are equal. Let us interpret this equal intensity specifically as indicating that any voter would be willing to give up his preference (to accept the reverse) for $100.00. Thus, passage of the legislation in question will benefit 51 per cent of the voters by $100 each, and it will harm 49 per cent of the voters by $100 each. In the hundred-man model, A would be selected by simple majority voting, and total benefits of $5100 exceed total losses of $4900.

Note that other voting rules need not produce this result, unless compensation of some sort is allowed. For example, under a 53 per cent voting rule the project could not be approved, and, in the additive sense employed above, the community would “lose” the potential $200 in benefits. However, if individual intensities of preference are not equal over all voters, this unique feature of simple majority rule disappears. If minorities feel more strongly on particular issues than majorities, then any rule short of unanimity may lead to policies that will produce net “harm,” even if the comparability of utilities among separate persons is still accepted as legitimate.

If vote-trading or compensation in any other form is allowed to be introduced, however, even this extremely restricted uniqueness of simple majority rule disappears. Let us continue to accept the equal intensity assumption. If compensation is introduced, any rule will cause A to be selected over 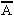in the foregoing example. If the unanimity rule were in force, for example, the 51 citizens who would be the potential gainers would have to compensate the 49 potential losers by at least $4900 in order to insure the passage of the legislation.*6 The demonstration that the same results would be produced under simple majority rule and the unanimity rule can be extended to apply also to less-than-majority rules. Suppose, for example, that we reverse the arithmetical model and consider the case in which 51 voters oppose the measure while 49 voters approve, and that each voter is willing to give up his preference for $100. If, in this situation, the community operates under a rule in which any person, individually, can order collective action, the potentially damaged majority will be able, out of the opportunity “benefit” they receive from not having the action taken, to fully compensate the members of the minority who might otherwise impose the change. Thus, even with equal intensities assumed from the outset, any voting rule will produce “desirable” results as measured by the comparative utility scales that are implicit in the assumption, provided only that compensation is allowed. However, if no compensation is allowed, either directly or through vote-trading, this restricted “welfare” conclusion no longer holds, and each rule must be analyzed anew for its welfare-producing properties.

As we have suggested, moral restraints may prohibit open buying and selling of votes. However, compensations may be arranged through vote-trading over a sequence of issues. If this is allowed to take place, the uniqueness of simple majority rule disappears, even on the equal-intensity assumption. The unique features reappear only when the equal-intensity assumption is extended to apply over all issues as well as over all voters. If all individual preferences are equally intense over a single issue, and if the preferences of each single individual are equally intense over all the separate issues in which he might participate as a voter, no vote-trading will take place (as we have shown above). Under these circumstances, and under these only, can simple majority rule be said to take on particular characteristic features that distinguish it from other decision-making rules.

Some of these points will be clarified in later chapters. The main purpose here is to emphasize the overly restrictive nature of the equal-intensity assumption. In our models we propose to place no such restrictions on individual preferences for the alternatives of political choice.

Equal Intensity and Random Variation of Preferences

The equal-intensity assumption may be employed, without great distortion, in the analysis of the situations in which the intensities of individual preference vary symmetrically among the separate and identifiable subgroups in the population and over all issues. In effect, this situation simply translates the equal-intensity case from the individual to the group level. This situation seems rather special. Normally, an act of government will either markedly harm or markedly benefit at least one specific and identifiable group which will, accordingly, feel more strongly about the issue than will the masses of voters. There are some measures undertaken by governments, however, which are relatively general in nature, that is, which apply in a relatively nondiscriminatory fashion to all individuals and groups. For such measures, individual preferences for and against may vary, but there seems to be no particular reason to expect that such variation would systematically reflect differential intensity. If this variation is distributed in some random fashion among all groups, the employment of the equal-intensity assumption may be reasonably appropriate.

Specific minorities on issues of this sort cannot readily arrange trades to secure favorable action. Majorities will tend always to be able to secure desired action under simple majority rule, and even under other rules if compensations are allowed. The constitutional calculus discussed in previous chapters is not changed significantly in application to this case. The decision-costs function might be changed somewhat, but the appropriate method of choosing decision rules is not modified. Insofar as the equal-intensity assumption is accepted as appropriate, the low-cost point on the aggregate “cost curve” would tend to be that represented by simple majority voting. If intensities of preference are assumed equal, anything desired by a majority, by sheer arithmetic, represents, when approved, a shift to the Pareto-optimality surface. The prevention of the implementation of the will of the majority, in this special case, is never to the “interest” of “society as a whole.” If simple majority rule is allowed to prevail, then “optimal” policy will always be selected.

This does not, of course, mean that majority rule will produce results that will be “optimal” for each individual in each particular case. In the case of equal intensity of preferences, the incremental payments that might be needed to obtain any qualified majority are simply transfer payments. The money would go from one man’s pocket into the next man’s, but there is no mutual gain from trade. In fact, there would be mutual loss when the costs of negotiating agreements are taken into account. Thus, at the time of constitutional choice, if an individual could feel confident that there would be a large number of such “equal intensity” issues to be put up for decision in the future, and if he felt that these issues would be such that his own position would fluctuate randomly between majority and minority without predictable differential intensity in the two cases, then he would expect any rule requiring compensation from the simple majority to a part of the whole of the minority to involve payments by him in some cases and payments to him by others in other cases. Over time, these could be expected to balance out. He might, therefore, wish to save himself the negotiating costs by accepting simple majority rule.

In order for this constitutional decision to be made, however, several conditions would be necessary. In the first place, there must be enough general (“equal intensity”) issues expected to arise to insure that they will, with respect to the individual, be mutually canceling. Secondly, the individual must feel fairly confident that he will not tend to be in the minority more than the average number of times. Thirdly, and most restrictive, there must be some method of distinguishing these “general” cases from those clearly characterized by differential intensities of individual preference. Little comment need be added on the first two conditions, but the third may be subjected to analysis. We might try two approaches: first, we might attempt to classify legislative activities that do not seem likely to generate differential intensities of preference among separate groups, and allow decisions on these activities to be made by simple majority rule; secondly, the constitution itself might be so designed that it automatically distinguishes among issues on this basis. The first approach is clearly feasible, and to some extent it is reflected in the constitutions of Western democracies.

Designing a constitution so that it will discriminate automatically between legislation potentially affecting intense minorities and legislation on which the intensity of desires is more or less equal, or can appropriately be assumed so, may not initially seem feasible, but this is, in fact, practicable. As discussed in Chapter 16, a properly designed bicameral legislature does make this distinction automatically.