Public Finance in Democratic Process: Fiscal Institutions and Individual Choice
By James M. Buchanan
Publisher
none
- Foreword
- Ch. 1, Introduction
- Ch. 2, Individual Demand for Public Goods
- Ch. 3, Tax Institutions and Individual Fiscal Choice
- Ch. 4, Tax Institutions and Individual Fiscal Choice
- Ch. 5, Existing Institutions and Change
- Ch. 6, Earmarking Versus General-Fund Financing
- Ch. 7, The Bridge Between Tax and Expenditure in the Fiscal Decision Process
- Ch. 8, Fiscal Policy and Fiscal Choice
- Ch. 9, Individual Choice and the Indivisibility of Public Goods
- Ch. 10, The Fiscal Illusion
- Ch. 11, Simple Collective Decision Models
- Ch. 12, From Theory to the Real World
- Ch. 13, Some Preliminary Research Results
- Ch. 14, The Levels of Fiscal Choice
- Ch. 15, Income-Tax Progression
- Ch. 16, Specific Excise Taxation
- Ch. 17, The Institution of Public Debt
- Ch. 18, Fiscal Policy Constitutionally Considered
- Ch. 19, Fiscal Nihilism and Beyond
 Earmarking Versus General-Fund Financing:
Earmarking Versus General-Fund Financing:
Analysis and Effects*
Introduction
In earlier models a
single tax institution finances a
single public service. Decisions were assumed to be made on the preferred quantities of public services, one at a time, and the costs of each service were assumed to be measured in one tax. The next step toward generalizing the model involves the modification of this restriction. Real-world fiscal structures are seldom characterized by segmented or assigned revenue sources to such a degree, although the institution of earmarked taxes is, relatively speaking, quite important, especially at state-local levels of government.
*19 Clearly, the institutional framework in this respect exerts some effects on individual behavior in fiscal choice, effects that should be subject to analysis.
The alternative to single-purpose, or dedicated, revenue sources for public services is, of course,
general-fund financing. In the one case, that of earmarking, the individual “votes for” designated taxes to finance specific public outlay. In the other, general-fund financing, he “votes for” the same taxes to finance, not a single service, but a budgetary bundle of several services, with the precise composition of this bundle being determined separately, presumably by some authorized budgetary authority. How will this single difference in fiscal institutions affect the individual? Which institution presents him with a more accurate basis for comparing alternatives? Will he tend to support greater over-all public outlay under earmarking or under general-fund institutions? And, if he is empirically observed to do so, what are the characteristic conditions that must be met?
Only one of these questions seems relatively easy to answer. If the individual can make separate fiscal choices for each public-goods program, which a structure of earmarked taxes conceptually allows him to do, directly or indirectly, he is informed as to the alternatives that he confronts, at least to the extent that the payment institutions allow, and subject, of course, to all of the qualifications noted in previous analysis. The uncertainty that he faces is clearly less than that which is present in the comparable decision on a “bundle” of public goods or services, with the mix among the separate components in the bundle to be determined in a separate decision process or through the auspices of a delegated budget-making authority. If this mix is not announced in advance to the voter-taxpayer, he must try to predict the outcome of another decision process, in which he may or may not participate, a process that need not exist at all in the more straightforward earmarking model where all revenue sources are specifically dedicated.
An earmarking system is closely analogous to that which normally confronts the individual chooser in private-goods markets, and, on several occasions, we have utilized the latter situation to assess the potentialities for rationality in individual behavior patterns. In private markets, the individual normally (although not universally) purchases one good at a time and separately; he makes a decision on, say, the amount of sugar per week that he plans to consume independently from his decision on the amount of gasoline or beer, although, of course, relations of complementarity and substitutability will exist. But only a fiscal system characterized by substantially complete revenue segregation would allow the individual, as a participant in political decisions, to attain a position comparable, even at a first approximation, to that which he confronts in private markets.
*20 General-fund financing is analogous to a market situation where the individual is forced to purchase a bundle of goods, with the mix among the various components determined independently of his own preferences. The specific tie-in sale is similar to general-fund financing, that is, nonearmarking. Making use of the theory of monopolistic tie-in sales in private markets, we can develop a theory of general-fund financing, and, conversely, a theory of earmarking.
*21
A Model of Individual Fiscal Choice
Consider a single individual as he confronts a fiscal choice situation. Collective services are available to the community at constant cost, whether supplied singly or jointly, and this cost is distributed among individuals in such a way that each person, also, faces a fixed supply-price, or tax-price. We may think of the goods as being financed by the levy of some invariant tax-price institution of the sort described early in Chapter 3; the individual has no power to influence his own “terms of trade” with the fisc. We also assume that the services or goods in question make up a relatively small share in the total income of the community, sufficiently so to allow us to neglect income effects in the analysis of individual behavior. We seek to analyze the behavior of the individual in selecting his most preferred outlay on public goods or services, recognizing, of course, that the model provides at best only some indication of voting behavior on spending proposals that are presented to the political group for decision.
As the earlier discussion has shown, the analysis is straightforward in the case of a single public good considered independently. We can derive an individual marginal evaluation schedule or curve, a demand curve in this instance, for the good in the same way that we derive such a schedule or curve for any privately marketed good or service. The supply curve confronting the individual, the tax-price curve, is a horizontal line at the fixed tax-price, which is some predetermined individualized share in the total community cost-price for the public good. Individual or private “equilibrium” is attained at the position where marginal evaluation or demand-price equals tax-price.
We seek to compare the results from this model with those from the contrasting one in which the individual is required to choose, not his preferred outlay on a single public good or service, but instead his preferred outlay on a bundle of two or more services, with the mix of components in this bundle being determined independently. For analytical simplicity, we shall develop this general-fund model in terms of only two goods. For descriptive flavor here, suppose that we are examining the individual’s choice for police-protection and fire-protection services in a municipality. The community supplies both services collectively, and we seek to determine the possible effects on individual choice behavior that may be produced by the two alternative budgetary systems. As indicated, general-fund financing introduces greater uncertainty to the extent that the individual cannot precisely know the content of the budgetary bundle. We want to leave this consideration out of account, however, and we assume that, prior to any conceptual voting process, the budgetary mix has been set and has been made known to all participants. Whether or not the individual has directly or indirectly participated in the determination of the mix is not relevant to our consideration. Given the mix as predetermined, the individual can predict with accuracy the allocation of tax dollars that are finally channeled through the budget. In this case, we try to answer the question: Will the individual vote for more or less public outlay than he would in the contrasting model where he conceptually votes on the two services independently? Will one or both of the two services in the mix be expanded by a shift from earmarking to general-fund budgeting?
The answers here depend upon the particular form that the budgetary tie-in takes. It would be possible to define this tie-in with respect to physical units of service, such as, for example, the requirement that one policeman be hired each time a fireman is hired, and vice versa. It seems descriptively more realistic and analytically more convenient, however, if we define the tie-in with respect to a budgetary allocation between the two services. In other words, general-fund financing takes the form of a specific proportion of the total budget assigned to each of the two services. There will exist one such budgetary allocation that will insure an identity of solution as between the two fiscal institutions, “solution” being confined, of course, to the individual calculus examined. That is to say, there will always be one budgetary ratio that will cause the individual to “vote for” the same relative quantities of the two services and the same total public outlay under general-fund financing that he would “vote for” under complete earmarking. This unique solution may be called “full equilibrium” for the individual, and this solution may be used as a starting point for the more extended analysis.
Geometrical Analysis
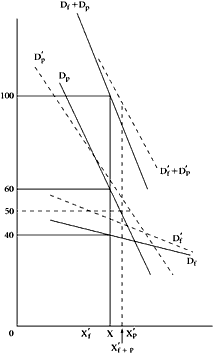 |
| Figure 6.1 |
It is convenient to develop the analysis geometrically. In Figure 6.1, quantity units are measured along the horizontal axis, but these are defined in a special manner. Under the tie-in arrangement, a unit of quantity is defined as that physical combination of the two services that are available for one dollar, one hundred cents. Thus, the total number of dollars expended is directly proportional to the distance along this axis. We begin by assuming that the “full equilibrium” budgetary mix prevails, and that this is defined as the forty-sixty ratio. That is to say, forty cents out of each budgetary spending dollar are allocated to spending for fire-protection services and sixty cents are allocated to spending on police protection. We can now derive demand curves D
f and D
p, respectively, for fire-protection and police-protection services. For analytical simplicity, we use linear relationships here, but this does not modify the results. These curves are derived with respect to the physical quantity dimensions indicated by the budgetary ratio. A single physical unit of fire-protection service is that quantity that is available, to the individual, at a tax-price of forty cents, and a unit of police service is that quantity that is available, to the individual, at a tax-price of sixty cents. (Note that this does not imply that the individual is able to adjust quantity privately, as in ordinary markets; the availability of a quantity to the individual at a tax-price implies only that this is the basis upon which he conceptually votes. Whether or not he actually secures these results depends on whether or not a sufficient number of his fellows agrees with him.)
The vertical summation of these two demand curves, D
f and D
p, yields the composite demand curve labeled D
f + D
p, which represents the demand for the bundle of the two services, mixed in the forty-sixty budgetary proportion. This is the bundle that the individual considers himself to be “purchasing” as he participates in collective or group choice under the general-fund scheme. By our definition of “full equilibrium,” this composite demand curve cuts the composite supply curve, drawn at one dollar, along the same vertical that measures the independently chosen quantities of fire protection and police protection. (There are elements of circularity in this geometrical construction, but these are not damaging to the analysis since its purpose is illustrative only.)
Under the conditions shown in Figure 6.1, there will be no differential effects as between earmarking and general-fund financing of the two services with the forty-sixty budgetary ratio. The individual will choose, will vote for, or otherwise use his political power to promote, the same quantity of services and the same over-all public spending under either of the two institutional forms. If separately presented, he would ideally prefer an amount, 0X, of fire-protection services, defined in forty-cent units, which can, of course, be readily translated into any other physical dimension. Similarly, he would ideally choose an amount, 0X, of police services, defined in sixty-cent units. Or, if he is forced to choose these two services combined in budgetary bundles, defined by the forty-sixty ratio, he will choose a quantity, 0X. In either instance, he will “vote for” a total budget outlay that is directly proportional to the horizontal distance, 0X, on Figure 6.1.
These two distinct fiscal institutions produce different results only when budgetary ratios other than that required for “full equilibrium” confront the individual in the general-fund scheme. To examine the differences, assume now that a segregated financing system has been in effect, but that a shift to general-fund financing at a fifty-fifty budgetary ratio is contemplated, with demand conditions remaining those depicted in Figure 6.1. To determine the effects of this change, it is first necessary to translate the two demand curves, D
f and D
p, into modified dimensions, the physical quantity units now being defined as those available, to the individual, at fifty cents. The new demand curves, drawn in the two fifty-cent dimensions, are shown as D’
f and D’
p. These are identical with D
f and D
p, except for the change in physical dimension. The effects of general-fund financing at this single nonequilibrium ratio, which now favors fire-protection services differentially, can be clearly shown. As common sense should suggest, more fire-protection services will be demanded in the tie-in arrangement and less police protection than would be the case under the segregated accounts. In the new quantity dimensions, 0X’
f represents the “full equilibrium” or earmarking quantity for fire-protection services, and, similarly, 0X’
p, the corresponding quantity for police services. In other words, these are the quantities in the new dimensions that correspond to 0X in the old dimensions. General-fund financing under the new, fifty-fifty, budgetary ratio will generate (as is indicated by the intersection of the new composite demand curve, D’
f + D’
p, and the composite supply- or tax-price curve at one dollar) a preferred tie-in quantity, 0X’
f + p.
*22
To this point, the conclusions are apparent. Any shift in the budgetary ratio away from that required for “full equilibrium” will insure that general-fund financing will introduce some distortion in the choice pattern of the individual. Forcing him to “purchase” two or more services in a bundle, rather than separately, will move the individual to some less-preferred position on his potentially attainable utility surface. Since, under independent adjustment for each service or good, the individual could, always, if he desired, select quantities indicated by the 0X”s in the new dimensions, the fact that he does not so do suggests that the new combination is less preferred than the alternative that is available under earmarking. The distortion causes him to desire that one of the two services be expanded beyond the “full equilibrium” amount and that the other be contracted to some quantity below this. Relatively, the good or service that is expanded will be that which is favored by the budgetary ratio. The analysis remains incomplete, however, until and unless further questions are answered. Will over-all public outlay, as desired by the individual, tend to increase or to decrease, and under what conditions? What are the characteristics of those goods and services most likely to be substantially increased as a result of favorable general-fund ratios?
The construction of Figure 6.1 suggests that total public outlay need not remain the same under earmarking and under nonearmarking when the latter embodies nonequilibrium budgetary ratios, and it also suggests that the direction of change may depend upon the particular configurations of the demand functions. Examination of the model produces the following conclusions: If the ratio turns in favor of the service characterized by the more elastic demand, at the full-equilibrium quantity (as is the case in the geometrical example here), total public outlay, as this is preferred by the individual, will be expanded by the shift from earmarking to the general-fund system. Conversely, if the ratio under general-fund financing favors the service characterized by the less elastic demand, again measured at the full-equilibrium quantity, total public outlay will be contracted by the shift in fiscal institutions. These specific results hold, without qualification, only for relatively limited shifts away from “full equilibrium” positions. Relative tax-price elasticities may change as the tie-in equilibrium changes. A more general conclusion is that total expenditure, as desired by the individual whose calculus we examine, will increase so long as the relative tax-price change, embodied in the budgetary ratio, is in favor of the service with the more elastic demand, with elasticity being measured at the respective tie-in equilibrium quantities of the two services. Conversely, total expenditure will fall if the ratio favors the service characterized by the lower tax-price elasticity of demand, similarly measured.
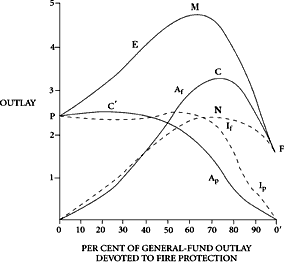 |
| Figure 6.2 |
Several of the relevant relationships are illustrated in Figure 6.2, which is based in the same underlying conditions as Figure 6.1. On the horizontal axis is measured the percentage of fire-protection outlay in a tie-in budgetary arrangement, running from zero to one hundred. On the vertical axis is measured total outlay, on both or on each service, as this is determined by the demand pattern of the individual and the assumed cost conditions. In all cases, as before, the quantities refer only to those preferred by the single individual whose decision process we analyze. As mentioned, Figure 6.2 is derived from the same date as Figure 6.1, which embodies linear demand functions, although a similar set of relationships could be readily derived from any postulated conditions of individual demand. The full-equilibrium ratio, defined previously as the forty-sixty one, must generate a desired or preferred total outlay under the tie-in that is equal to the sum of the preferred outlays on the two services when they are “purchased” separately, through an earmarked revenue arrangement. If a budgetary ratio with 0 per cent outlay on fire protection is introduced, total outlay will be exclusively on police. Conversely, if a 100 per cent ratio prevails, all outlay is on fire protection. Hence, if income effects are neglected, the vertical distance, E, at the forty-sixty ratio, must be equal to the sum of the distances, 0P and 0’F.
As the ratio shifts from the forty-sixty position in favor of fire-protection services, desired total outlay on both services in a tie-in bundle expands, as shown by the rising portion of the top curve in Figure 6.2 to the right of E. As this shift continues, preferred total spending increases until it attains a maximum at M, after which it falls sharply to F. As the ratio shifts from the forty-sixty position in the other direction, now differentially favoring police services, desired total outlay on the tie-in bundle falls, as shown by the top curve to the left of E. It continues to fall to point P, where no part of the budget is allotted to fire protection.
The lower four curves in Figure 6.2 break down this preferred total outlay as between the two services and as between actual and imputed components. The actual outlay on one service is readily computed by taking the indicated percentage of total outlay as shown by the ratio on the horizontal scale. The two actual outlay curves must, of course, sum to the combined outlay curve for the bundles, and the two curves must intersect at the 50 per cent position. “Imputed outlay” on a service is defined as that part of preferred total outlay on a bundle containing that service that is attributed by the individual to that service at each particular tie-in equilibrium. Imputed outlay on a service equals actual outlay only at the full-equilibrium budgetary ratio and at either extremity of Figure 6.2. For all other ratios, imputed outlay differs from actual, and the difference reflects the degree of “exploitation,” negative and positive, that nonequilibrium budgetary ratios generate. Imputed outlay falls below actual outlay on the service that is favored in the budgetary mix; it exceeds actual outlay on the remaining service. This is shown in Figure 6.2. To the right of 40 per cent, imputed outlay on fire-protection services, I
f, lies below the curve of actual outlay, A
f. To the left of 40 per cent, the opposite relationship holds. And, of course, the relationship for police services is the inverse of those for fire protection since the two imputed outlays must also equal total outlay on the combined bundles.
*23
Maximum total outlay is reached at M. As the ratio shifts beyond this point, desired total expenditures fall although the share of this total devoted to fire protection continues to rise. At some point, C, to the right of M, these two factors become mutually offsetting, and some maximum outlay on fire protection alone is attained. Increasing the share in a combined budget beyond this “critical ratio” will result in fewer resources being devoted to fire protection, always on the assumption that the desires of the voter-taxpayer-beneficiary whose calculus is here examined imply something about collective outcomes.
As the ratio shifts differentially to favor police services, in the model used here, total outlay falls continuously. However, because of the increasing budgetary share, the preferred quantity of police services increases to some critical ratio, C’, where actual outlay on this service alone reaches a maximum.
*24
Implications for Fiscal Choice
The analysis demonstrates that the differential effects of earmarking and general-fund financing depend critically on the way in which general-fund budgetary arrangements allocate revenues among the several public services or goods in the bundles that are presented for choice. This suggests the question: What predictions can be made, if any, concerning the composition of a general-fund budget? To the extent that a budgetary authority is assumed able to make decisions on the mix in complete independence of the preferences of citizens, no predictions are possible. However, if we look at a slightly different question, some interesting predictions can be made, and, in this way, the broad effects of these alternative fiscal institutions can be provisionally traced. If earmarking and general-fund financing, as institutions, are alternatives, we may predict something of the effects if we know something about the choice among these alternatives. Under what conditions is a community more likely to shift from earmarking to general-fund financing and vice versa? What responses to group pressures do such shifts reflect?
In any politically organized community, specific individuals and groups will find particular interest in promoting the performance of one or the other of the public services that are provided. Using our two-service model to be illustrative of the more general case, the predicted behavior of these separate groups can be examined. Once this is done, inferences can be drawn concerning the impact of the institutions on fiscal choice patterns. Assume that both of the services are financed initially through earmarked revenue sources; choices are made independently. It is clear from the analysis above that groups organized in support of either service would have incentives to push for a shift to general-fund financing,
if differentially favorable budgetary ratios can be expected to result. Both groups cannot, however, simultaneously hope to secure such favorable ratios, unless one of them makes gross errors in predicting political responses. The characteristics of demand make for significant differences in the expected gain to be secured from favorable general-fund schemes. Relatively, the group that is organized in support of the more elastic-demand service stands to gain more by favorable tie-ins. Sizeable amounts of “taxpayers’ surplus” can be captured from the relatively less elastic-demand service that is tied in under a budgetary bundle.
*25 Not only will the favored service be allotted a larger share of each spendings dollar, but, also, total spending on both services increases. The group organized in support of this service can, therefore, afford to take some risk of unfavorable budgetary ratios in general-fund schemes. By comparison, the pressure group supporting the extension of the relatively inelastic-demand service, will not be able to secure so much advantage from comparably favorable shifts in the tie-in ratio. While a higher share of each budget dollar will be desirable, the potential “taxpayers’ surplus” that is exploitable is severely limited. This group stands to gain less and probably to lose more from a change in institutions toward the amalgamation of revenues. It will, therefore, tend to opt for the continuation, or the introduction, of segregated budget accounts.
The hypothesis that emerges is that shifts toward general-fund fiscal arrangements tend to be made in response to pressures from those in support of services that will be benefited most from such arrangements. If this hypothesis is valid, and if the political response is as these groups predict, institutional changes away from earmarking produce somewhat larger public expenditures in total. This general conclusion is reinforced by the behavior of explicitly organized taxpayer groups and by that of the bureaucracy. Taxpayer associations, organized for the purpose of holding down tax rates, independently from any consideration of public service benefits, seem likely to support the retention of earmarked revenues. By comparison, the organized bureaucracy, whose interest is diametrically opposed to those of the taxpayers, and which is interested in expanding the size of the public sector, independently of cost considerations, seems likely to support general-fund financing. This effect is over and above the specific budgetary objective of maximizing the power of the budgetary authority, which, of course, supports general-fund schemes for even more obvious reasons.
These generalizations need not, of course, apply in all cases, and empirical tests may refute the basic hypotheses that are suggested or implied by the analysis. Whether or not earmarking or general-fund financing provides, in the large, the more “efficient” fiscal institution, from the individual’s own frame of reference, has not been considered. The analysis has not taken into account the costs of making political decisions, and, when these are included, general-fund financing becomes relatively more attractive, as an institution, because of the reduction in these costs that it makes possible. Considerations of this nature are put aside in this part of the study since we have assumed that the institutions are selected externally to the individual.
Numerical Appendix to Chapter 6
The geometrical construction in Figures 6.1 and 6.2 is drawn to scale from the numerical model explained here.
I. Construct two linear demand equations, one for fire-protection services, one for police-protection services. These equations are of the standard form:
y =
a –
bx, but the following side conditions must be satisfied.
y
f = 40 and
y
p = 60, when
x
f =
x
p.
These conditions are imposed by the definition of “full equilibrium” at the 40-60 budgetary ratio.
II. Define the demand equation for fire-protection services as
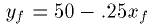 |
(1) |
Solving this equation for
x
f , when
y
f = 40, we get,
x
f = 40.
Now derive a demand for police-protection services that satisfies the side conditions in I, as follows:
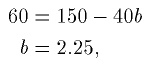
giving
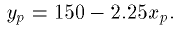 |
(2) |
III. Equations (1) and (2) are demand equations for the two services defined in quantity units as follows:
Fire protection—one unit is “the physical quantity available to the individual for a cost-price of forty cents.”
Police protection—one unit is “the physical quantity available to the individual for a cost-price of sixty cents.”
IV. From (1) and (2) construct a composite demand equation (3),
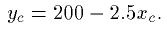 |
(3) |
This is the demand equation for the one dollar “bundle” of services, combined in the 40-60 budgetary ratio.
V. At “full equilibrium,” compute
total outlay on both fire-protection services and police-protection services. Compute
actual outlay on each service, and also
imputed outlay on each service. These results are entered in Table A-6.1, opposite the 40-60 budgetary ratio. Note that actual outlay in this solution must equal imputed outlay for each service, by definition of the full equilibrium condition.
with Demand Equations as Defined; Figures Rounded Off |
|||||
|
|
|||||
| Budgetary Ratio Fire to Police in General-Fund Budget | Total Outlay | Actual Outlay Fire Protection | Inputed Outlay Fire Protection | Actual Outlay Police | Imputed Outlay Police |
|
|
|||||
| 00-100 | 2400 | 0 | 0 | 2400 | 2400 |
| 01-99 | 2429 | 24 | 31 | 2405 | 2398 |
| 10-90 | 2707 | 271 | 325 | 2436 | 2382 |
| 20-80 | 3079 | 616 | 711 | 2463 | 2368 |
| 30-70 | 3516 | 1055 | 1145 | 2461 | 2371 |
| 40-60 | 4000 | 1600 | 1600 | 2400 | 2400 |
| 50-50 | 4487 | 2244 | 2010 | 2243 | 2477 |
| 60-40 | 4795 | 2877 | 2299 | 1918 | 2496 |
| 70-30 | 4709 | 3296 | 2402 | 1413 | 2307 |
| 80-20 | 4000 | 3200 | 2400 | 800 | 1600 |
| 90-10 | 2826 | 2543 | 2169 | 283 | 657 |
| 99-01 | 1712 | 1695 | 1652 | 17 | 60 |
| 100-00 | 1600 | 1600 | 1600 | 0 | 0 |
VI. Change the budgetary ratio from that defined as “full equilibrium.” Take a new ratio, 50-50.
Change the
quantity dimensions in the two demand equations as appropriate to derive
translated functions (1)’ and (2)’,
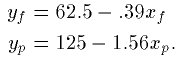 |
(1)’ (2)’ |
VII. From (1)’ and (2)’, derive new composite demand equation,
 |
(3)’ |
This becomes the demand equation for the one dollar “bundle” represented by services combined at the 50-50 ratio.
VIII. Solve (3)’, for
x
c, when
y
c = 100, which is the “cost-price” of the “bundle,” and get,
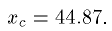
IX. Now solve (1)’ and (2)’ for
y
f and
y
p, when
x
f =
x
p = 44.87. Get
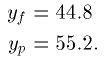
X. From the values derived in VIII and IX, derive
actual and
imputed outlay on each service and
total outlay on both services and insert results in Table A-6.1. These values are derived as follows:
| Total outlay | = (44.87) (100) | = 4487 |
| Actual outlay on fire-protection services | = (44.87) (50.00) | = 2243.50 |
| Imputed outlay on fire-protection services | = (44.87) (44.80) | = 2010.18 |
| Actual outlay on police-protection services | = (44.87) (50.00) | = 2243.50 |
| Imputed outlay on police-protection services | = (44.87) (55.20) | = 2476.82. |
Note that total outlay must equal sum of the two actual outlay figures and also the sum of the two imputed outlay figures.
XI. Compute outlay figures for all remaining budgetary ratio in a similar manner and insert results as appropriate in Table A-6.1.
Journal of Political Economy, LXXI (October, 1963), 457-69, circulated also as No. 73, Studies of Government Finance Reprints, Brookings Institution, 1963.
Earmarked State Taxes (New York: Tax Foundation, 1965).
Review of Economics and Statistics, XLII (February, 1960), 68-73; “A Theory of Full-Line Forcing,”
Northwestern University Law Review, LV (1960), 62-95. See also Ward S. Bowman, Jr., “Tying Arrangements and the Leverage Problem,”
Yale Law Journal, LXVII (March, 1957), 19-36.
p curve at the left of Figure 6.2 requires some explanation. As the budgetary mix shifts in favor of police services, imputed expenditures on both services fall, as shown. However, as a smaller and smaller share of the budget is allotted to fire protection, the degree of exploitation that consumers of police services can attain reaches some maximum. Beyond this point, the “relative tax-price reduction” that the tie-in involves is progressively diminished.
The derivation of the construction is clarified in the numerical example upon which the figures are based, which is presented in the Appendix. It should be emphasized, however, that the general results do not depend on the particulars of the example or on the shapes of the curves derived therefrom.
Review of Economics and Statistics.
The analysis of general-fund financing, developed here, is simpler than the comparable analysis of monopolistic tie-in sales. In our model, the unit cost of supplying services, either jointly or separately, is always equal to the tax-price charged to “purchasers.” The government does not seek to make profits. With the monopolist, the difference between unit cost and price is a central variable that the fiscal model need not include.

